Practically all phenomena of classical physics are governed by partially derivative equations (PDE).
In most cases they are of the second order,


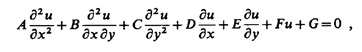
where A to G are constant coefficients.

Here we see the equation of two independent variables x and y, but usually the number of variables is four – space variables x, y, z and time t.

Unknown function u = u(x, y, z, t), in general, depends on space variables x, y, z, and on time t. Sometimes equation doesn’t depend on time t (stationary problems), sometimes only 2-dimensional problems are considered (u depends only on two space variables x, y) or only 1-dimensional problems (u depends only on one space variable x).

To solve the equation, usually initial conditions (appearance of function u for t=0) and boundary conditions (appearance of function u or some derivative of this function on the boundary of the region where we solve the equation) are defined:

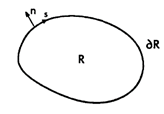
Boundary conditions are specified in three ways:

Three categories of PDE can be distinguished (each category is defined only by the first three coefficients A, B, C – coefficients corresponding to the second order derivatives). These are:



If the coefficients, A to G, are functions of x, y, z, then this classification can still be used if A, B and C are given a local interpretation. This implies that the classification of governing equations can change in different parts of computational domain.

For instance, for 2-dimensional steady compressible potential flow about a slender body the governing equation is

This equation is elliptic for subsonic flow (M < 1) and hyperbolic for supersonic flow (M > 1)).

The situation, when equation change its category, are usually more complicated for calculations. For instance, it is difficult to calculate an air flow, when aircraft is crossing the sound barrier.

Below we consider more simple cases where coefficients A, B, C, …, G are constants and thus the category of PDE is fixed.

1. Hyperbolic equations

   The typical example of hyperbolic PDE is the wave equation.
   Learn more…

2. Parabolic equations

   Parabolic PDE appears in transferring problems, where some mechanisms of dissipations exist, for instance, considering viscous fluids or considering problems of thermal conductivity.
   Learn more…

3. Elliptic equations

   Elliptic PDEs are usually associated with steady-state problems.
   Learn more…